THE IMPACTING INVERTED PENDULUM
General Considerations
The inverted pendulum is used in the modelling of
several engineering applications, such as
rings, printers, machine tools, dynamics of rigid standing
structures, mooring buoy, moored vessels in a harbour against stiff
fenders, rolling railway wheelset. Impact oscillators have also applications in the
emerging field of micro electro mechanical systems (MEMS).
Furthermore, one prosiming application is in the field
of impact dampers, which are nowadays used in several fields,
including mechanical and civil applications, to reduce high-amplitude
vibrations of a principal structure to be protected.
The mechanisms of protection consist in energy dissipation or in
frequency shifting, and impact dampers are sometimes preferred to
classical viscous dampers due to their simplicity, reliability, and
easyness of maintenance. Examples of structures which can be safely
protected by impact dampers are cutting tools, turbine
blades, tall flexible structures like
chimneys, antennas, traffic-lights, scaffolding: here the impact
damper is successfully used to suppress high frequency modes caused
by vortex shedding.
The use of a passive impact damper is limited due to its
ineffectiveness for broadband excitation. To overcome this
limitation, control of the dynamics of impact dampers has been
proposed, both by on-line control algorithms or by a
"on-off" control technique. Also control of chaos
techniques, exploiting the nonlinear characteristics of the dynamics,
have been applied.
An impact damper based on the inverted pendulum is able to overcome
the previous drawback by a passive mechanism. In fact,
its main characteristic is that it has no internal frequencies, so it
can work well, at least in principle, on a broad range of frequencies.
Another distinguished characteristic is that it has an activation
threshold before it starts to dissipate, below which the pendulum rests
on the lateral barrier. Accordingly, if properly tuned, it starts to
work only when the vibrations of the main structure overcome a
certain threshold, namely, it (automatically) activates only when
necessary.
The previous properties are very useful in many applications, and call for
an extended analysis of the dynamical response of the systems, which is
the main objective of the present work. However, this not the unique
motivation for this analysis. In fact, the inverted pendulum represents
an archetype of a family of impacting oscillators whose dynamics
possesses some specific phenomena, such as chattering and
multistability, which are of theoretical interest per se.
It is worth noting that, due to the negative stiffness, the well
known "grazing" bifurcation, due to a "soft" approch
of the mass toward the walls, is not common for this softening
non-smooth systems, while being characteristic of hardening
non-smooth systems. Indeed, it has been observed only
in few, very specific pathological situations, not of interest for the
present work. The role of grazing in characterizing the non-smooth response
is here assumed by chattering.
Results
A systematic numerical investigation of the nonlinear dynamics of a
simple impact damper has been performed.
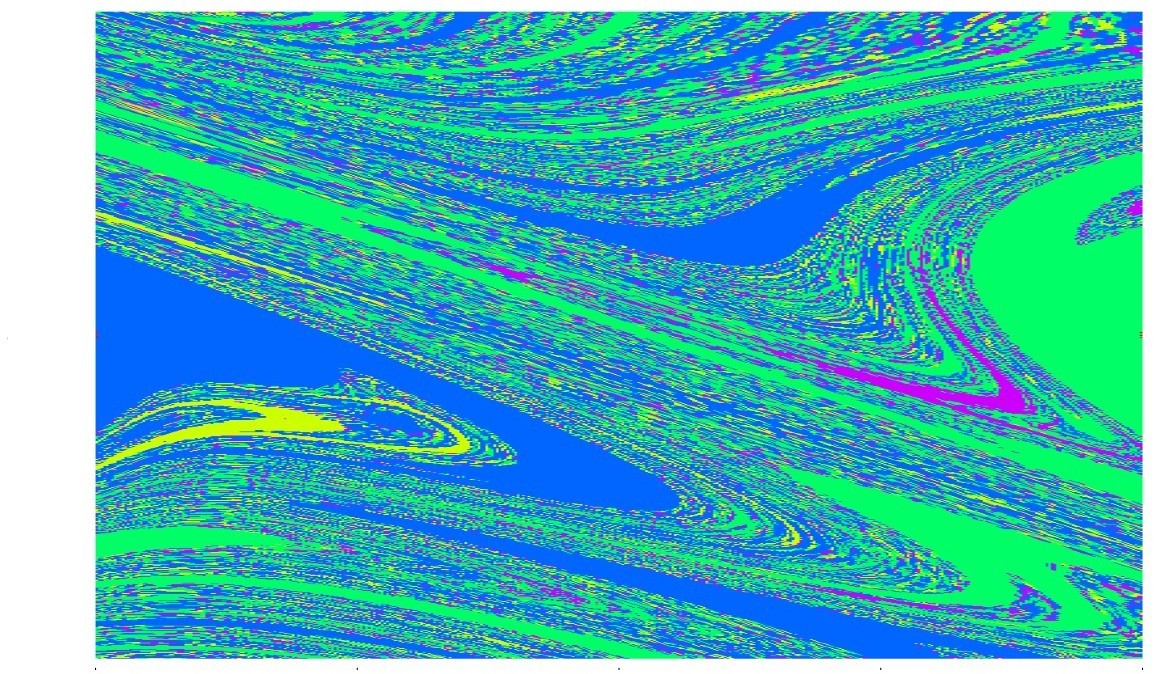
The periodic attractors scenario has been considered first. It has
been determined by studying the range of existence of the main stable
cycles by the combined use of numerical basins of attraction and
bifurcation diagrams. Attention was focused on local and global,
classical and non-classical bifurcations that lead to the
attractors-basins metamorphoses.
The periodic attractors map has been discussed and illustrated in
detail, and it has been shown how in a certain region of the
parameters space the multistability of several periodic attractors,
of various period and both confined and scattered, is common, while
in other regions only one cycle is observed. This provides a large
flexibility in view of practical applications, permitting to choose
different regions of behaviour according to required different
performances.
The chattering appearing just at the end of the rest position, which
is the main characteristic non-smooth phenomena of this class of
non-resonant oscillators, was then investigated. Some attempts have
been made to estimate the time length of chattering, which also
provides information on the disappearing of chattering for increasing
values of the excitation amplitude.
Finally, the occurence of robust chaotic attractors, the third
class of observed attractors, has been studied. Two different
qualitative behaviours, one for small and one for large frequencies,
have been observed and described in detail. The transition from
confined to scattered chaotic attractors through a boundary crisis
related to the homoclinic bifurcation of the hilltop saddle has been
illustrated by an example.
Various developments of the present investigation can be sought and
appear to be worthy. Among others, we quote a better understanding of
the chattering behaviour, possibly by using abstract analytical
tools, and the extension to more generic excitations,
which are more realistic and arises in the field of chaos control.
-
S. Lenci, L. Demeio and M. Petrini, Response scenario and non-smooth
features in the nonlinear dynamics of an impacting inverted pendulum,
Accepted for publication in the Journal of Computational and Nonlinear
Dynamics, April 2005.
-
S. Lenci, L. Demeio and M. Petrini, Some aspects of the non-smooth
dynamics of an impacting inverted pendulum,Proc. 5th Euromech
Nonlinear Dynamics Conference, ENOC-2005, Eindhoven,
The Netherlands, August 7-12 2005 (submitted).

